SIAM News

Dune Dynamics at DS ’07

Volume 40, Number 9, November 2007

By Barry A. Cipra

The answer, my friend, is blowin’ in the wind.—Bob Dylan

Sand dunes are ubiquitous, sometimes problematic features of deserts and coastlines. Their undulations call to mind ripples on water, but dunes and water waves differ in crucial ways. In particular, whereas “traveling” water waves are largely illusory, a product of motion that is essentially up and down, the advance of sand dunes really does involve material transport: Individual grains of sand are blown by the wind along nearly horizontal trajectories, as anyone whose face has been sandblasted at the beach on a breezy day will know. Errant dunes have been known to block roads and overrun buildings.

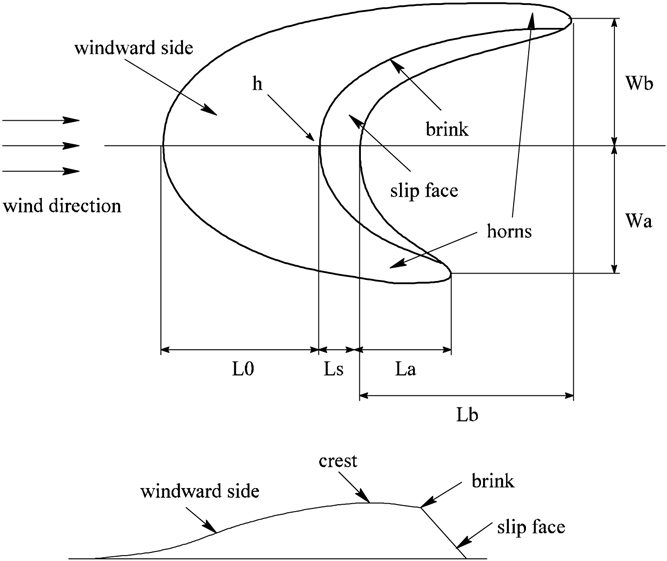
Figure 1. Anatomy of a dune. Forces exerted by wind, gravity, and friction determine the
shape of a barchan. Figure courtesy of Hans Herrmann.

Because of how they move, sand dunes also differ from water waves in shape. Of particular interest is a class of crescent-shaped dunes known as “barchans” (see Figure 1, top). In an invited address at this year’s SIAM Conference on Applications of Dynamical Systems, held in Snowbird, May 28 to June 1, Hans Herrmann of ETH Zürich described analyses he and colleagues have done of these fascinating formations.

The seminal scientific study of sand dunes, The Physics of Blown Sand and Desert Dunes, by Ralph Bagnold, dates back to 1941. In his book, Bagnold provided a Goldilocks-like classification for the aeolian (wind-driven) transport of sand. Sand that’s too coarse creeps along the ground; sand that’s too fine stays in suspension, blowing great distances. In the “just-right” phase, corresponding to grain diameters between 0.1 and 0.3 millimeters, sand moves by a process called “saltation,” from the Latin verb saltare, meaning “to leap.”

In saltation, the impact of a given sand grain transfers momentum to other grains, which follow ballistic trajectories accelerated by the wind until they too hit the surface, kicking up other grains in turn. Because some kinetic energy is lost in the (inelastic) collisions, saltation dies out if the wind speed is too low, but it grows exponentially if the wind speed exceeds a certain threshold (see Figure 2). The latter case is selflimiting: Blown sand sucks energy out of the wind, eventually reducing its speed.

Saltation, Bagnold found, is the dominant mechanism in the formation of all sand dunes. The dunes will be barchans when wind blows mainly from one direction over largely flat terrain with relatively little sand. (When sand is abundant, formations known as transverse dunes are the rule.) Sand builds up on the backside of an incipient bump and slips around the sides to form a pair of arms reaching downwind. The build-up near the crest eventually reaches an unstable slope on the downwind side known as the angle of repose, which for sand is roughly 34 degrees. Sandslides leave the dune with an axial profile consisting of a shallow curve on the upwind side and a steep straight line downwind (see Figure 1, bottom).

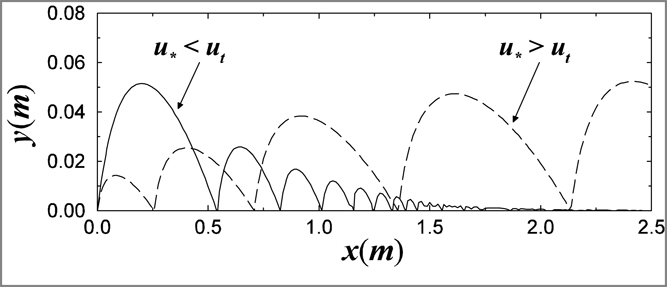
Figure 2. Saltation dies out if wind speed is too low (solid curve), but becomes self-sustaining
for winds in excess of a critical threshold (dashed curve). Figure courtesy of Hans Herrmann.

Barchans typically appear in clusters, often made up of thousands of separate dunes of various sizes. Much like glaciers, barchans appear to be stationary but in fact advance slowly, on the order of centimeters per day. (Unlike glaciers, though, the physical transport of sand grains takes place only in a surface layer; a given grain travels in fits and starts, going great distances in a matter of days before being stochastically buried for years, decades, or centuries.) Small dunes, it’s been observed, travel faster than large ones. This begs the question, What happens when a small barchan overtakes a larger one?

People have not been measuring sand dune migration long enough to have observed the dynamics of interacting dunes—no ancient astronomers of sand kept meticulous records of barchan vagaries. Modern researchers have proposed various explanations, ranging from simple, permanent coalescence to soliton-like behavior in which the small dune merges momentarily with its bigger brother, only to reemerge in its original size and shape on the other side.

Herrmann and colleagues, including current and former students Gerd Sauermann, Veit Schwämmle, and Orencio Durán, have spent much of the current decade bringing computational precision to the study of dunes. They have extended the earlier analytic models of sand physics, developed computer models to solve the equations of shape and motion, and done field experiments with dunes in Morocco and Brazil, among other locations. Their in silico barchans look a lot like the in silica originals.

An obvious starting point for analyzing sand flow is conservation of mass: The piling up or erosion of a dune at any point—i.e., the time derivative of dune height—is proportional to the gradient of the flux. The latter is a function of wind speed (itself a function of height) and the dynamics of saltation, which includes coefficients of drag and restitution. Because all motion occurs at the surface, the units of flux are kilograms per meter per second, rather than kilograms per meter squared per second; the constant of proportionality is the density of sand in the mobile surface layer.

Bagnold derived a simple formula for the flux, finding it to be proportional to the cube of the wind friction speed u, for speeds in excess of the threshold speed u_t, which is about a third of a meter per second. (Wind friction speed is derived from the vertical profile of wind speed, which depends on surface roughness.) In the 1970s, Heinz and Katharina Lettau of the University of Wisconsin modified Bagnold’s formula to make flux proportional to u²(u – u_t). Herrmann’s group has derived a yet more complicated formula based on a closer look at the details of saltation. Their formula still has flux asymptotic to u³ for large u, but takes a different form for speeds just above the threshold and gives results that, after parameter fitting, agree well with wind tunnel experiments.

Using their equations and the commercial code FLUENT, Herrmann and colleagues have simulated barchan formation and motion. The computations generate the graceful crescent shapes of observed dunes and reproduce their relative migratory speeds. In a 2003 paper in Nature, Herrmann and Veit Schwämmle described soliton-like behavior when a smaller barchan catches up with a larger one. In further work, Herrmann and Durán found a range of behavior, depending on the ratio of dune volumes (dividing the smaller by the larger). Solitonic pass-through occurs when the ratio is larger than about 0.25. For ratios under 0.07, the smaller barchan is simply swallowed as it climbs the backside of the larger one. Between 0.07 and 0.14, the smaller dune emerges as multiple barchanettes, a process the researchers call “breeding.” From 0.14 to 0.25, the smaller dune splits in two, as if “budding” off the horns of the larger barchan (see Figure 3).

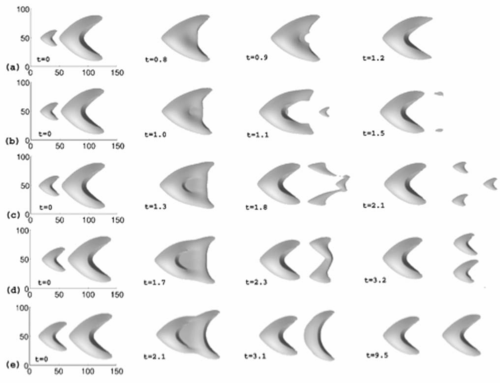
Figure 3. Simulations show various possible outcomes when a smaller barchan overtakes a larger one, depending on their relative sizes: coalescence (a), “breeding” (b and c), “budding” (d), and solitary wave behavior (e). The height of the larger barchan is 5 meters; the heights of the smaller barchans are 1.8, 1.9, 2.2, 2.6, and 3.1 meters, respectively. Time is given in
months. From “Breeding and Solitary Wave Behavior of Dunes,” by Oscar Durán, Veit Schwämmle, and Hans Herrmann.

The speed at which a barchan moves, Bagnold found, is inversely proportional to its height. That would seem to imply a nonsensically nearinfinite speed for nano-barchans. In fact, barchans below a certain critical size are simply not seen. Herrmann, Durán, and Eric Parteli have shown that the barchan-forming threshold depends on the wind speed and the flux of sand in the area between dunes: As the values of these variables increase, the threshold drops. (Intuitively, this makes sense: A dune cannot move faster than the wind that carries the sand.)

Herrmann and Durán also recently derived a set of differential equations describing the competition between dune mobility and plant growth. Vegetation tends to retard erosion (mainly by slowing down the wind) and to persist until deposition buries (and kills) it. Plants take root most easily where the erosion/deposition rate is small to begin with. For barchans, this includes the horns of the crescent. As a consequence, the horns are pinned by plants while the crest between them advances, until the barchan inverts into an upwind-pointing parabola. Herrmann and Durán’s equations offer insight into the salta-tion/vegetation battle. According to simulations based on their model, for example, inversion occurs only when the (dimensionless) ratio of characteristic erosion rate to vegetation growth velocity is smaller than about 0.5.

In another recent paper, Herrmann, Schwämmle, Durán, and Pedro Lind considered the dune size and spatial distributions in barchan fields. Their key equation, derived by a mean-field approach, relates the characteristic interdune spacing L to the average dune width W and the standard deviation S of the distribution of widths: L is proportional to S³/W². In other words, the sparser the dune field, the greater the variability in dune size. (The size distribution itself is found to be log normal.)

Not unique to Earth, sand dunes are also abundant on Mars. Many of the crucial constants— gravity, wind resistance, and grain size—are different, but the physics is otherwise the same. In a detailed analysis, Herrmann and Parteli showed that saltation is an order of magnitude more sensitive to wind speed increments on Mars than on Earth. The strength of Martian gravity being about 38% of that on Earth, a kicked-up grain is accelerated by the wind roughly seven times longer than an equivalent grain on Earth. When feedback effects are included, Martian sand grains attain ten times the speed of their terrestrial counterparts. The researchers have found that certain observed dune fields are consistent with wind friction speeds of about 3 meters per second. Other fields, they’ve found, can be explained (in their model) only under the assumption of a bimodal wind regime, with the wind alternating between two directions (see Figure 4).

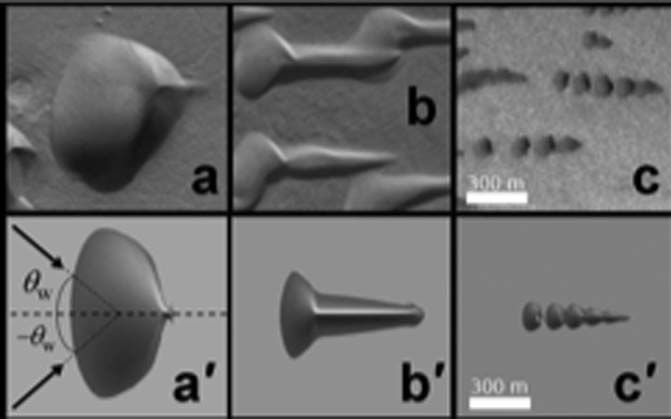
Figure 4. Alternating explanations. Unusual dunes on Mars (top) can be reproduced in simulations by assuming an alternating wind field (bottom). From "Saltation Transport on Mars," by Eric Parteli and Hans Herrmann. (Martian photos taken by the Mars Orbiter Camera, courtesy of NASA/JPL/MSSS.)

The study of dunes, Herrmann points out, involves all three components of modern science: theory, computation, and experiment. Field measurements, he says, are vital. Given where dunes tend to be found, experiments are also hot, dirty, grueling work. Herrmann recalls an urgent call from Parteli’s mother, shortly after Parteli returned from a two-week trek through South America, begging Herrmann not to send her son on the next field trip. Parteli, it seems, had mentioned that for his next assignment he would be studying the dunes on Mars.

Barry A. Cipra is a mathematician and writer based in Northfield, Minnesota.